Power Perpetuals

Introduction

This paper introduces a new type of derivative, the power perpetual.

If the price of ETH doubles, the ETH^2 power perp 4Xs, the ETH^3 power perp 8Xs, and the ETH^5 power perp 32Xs.

Of course, this asymmetric upside doesn't come for free. Those who are long a power perpetual must regularly pay a premium yield to those who are short it.

Power perpetuals provide global options-like exposure without the need for either strikes or expiries, giving them the potential to consolidate much of options market liquidity into a single instrument.

In many ways, power perpetuals are the logical next step from Everlasting Options, and they have been independently discovered at least two other times to our knowledge, by researchers Wayne Nilsen and llllvvuu.

Mechanism

Prerequisites

Power perpetuals are a particular family of the class of perpetual derivatives introduced in the Everlasting Options paper.

We will assume familiarity with both Everlasting Options and the basic mechanics of perpetual futures for the rest of this paper.

Definition

A power perpetual is a perpetual derivative indexed to a power of the price of some underlying instrument. In this paper, we will assume this underlying instrument is Ether.

For any power p, the ETH^p power perpetual is kept in line by means of a funding fee paid at a regular internal, say, daily. If the current price of the Power Perpetual is $MARK at the time of funding, those who are long the Power Perpetual may pay those who are short $(MARK-INDEX) = $(MARK-ETH^p).

In the context of power perpetuals, we call this funding fee a premium yield to reflect that this cost generally represents premium being paid from longs to shorts in return for options-like exposure.

Example

Consider the ETH^2 power perpetual.

Assume for the sake of simplicity that ETH is trading at $3, and that the ETH^2 power perpetual is trading at $9.09 at the time funding is paid. Then the longs would have to pay the shorts $(MARK-INDEX) = $(MARK-ETH^2) = $(9.09 - 3^2) = $9.09 - $9.00 = $0.09 per contract.

Pricing

Overview

Power perpetuals with powers greater than one have positive convexity, meaning holders make money faster as prices move in their favor and lose money more slowly as prices move against them. In the language of options, we would say they have positive gamma.

Just as an option generally trades at a premium to its intrinsic value, the ETH^p perp generally trades at a premium to the _p_th power of the price of ETH.

Derivation

Following the methodology in the Everlasting Options paper, we can start by pricing an expiring power derivative, then price a portfolio of these derivatives equivalent to the desired perpetual.

We will use Black-Scholes assumptions to derive our price below. These are certainly not the most appropriate assumptions, but should serve as an example of how a market-maker might go about valuing a power perpetual.

Pricing an expiring power derivative under Black-Scholes assumptions is significantly simpler than pricing an option. Those curious can find a quick derivation on StackExchange here. The price comes out to

where S is spot price, p is the power, t is the time to expiry, r is the drift or risk-free rate, and v is the annualized volatility.

Combining that with the pricing methodology for Everlasting Options found in Appendix B of the Everlasting Options PDF and summing the resultant geometric series, we obtain the following expression for the price of a power perpetual with funding paid once per period (assuming the series converges — see below):

where f is the funding period in years.

This can be interpreted as the index, , times an adjustment factor, which accounts for the embedded optionality in the power perpetual. Note that, as the funding period approaches 0, this adjustment factor approaches 1.

The premium yield (our new term for funding rate) can be calculated as

Convergence

Unlike stock Everlasting Options, which we can always price, poorly formulated power perpetuals may have prices which fail to converge. In particular, we can only price a power perpetual when we have

Intuitively speaking, the higher the power and volatility, the more valuable longer-dated expiring power futures get, and the longer the funding period, the more of the value of the power perpetual is concentrated in longer-dated power futures. For some combinations, the equivalent portfolio can become infinitely valuable.

This problem can easily be avoided in practice by choosing a sufficiently small funding period.

Examples

ETH^2 Power Perp

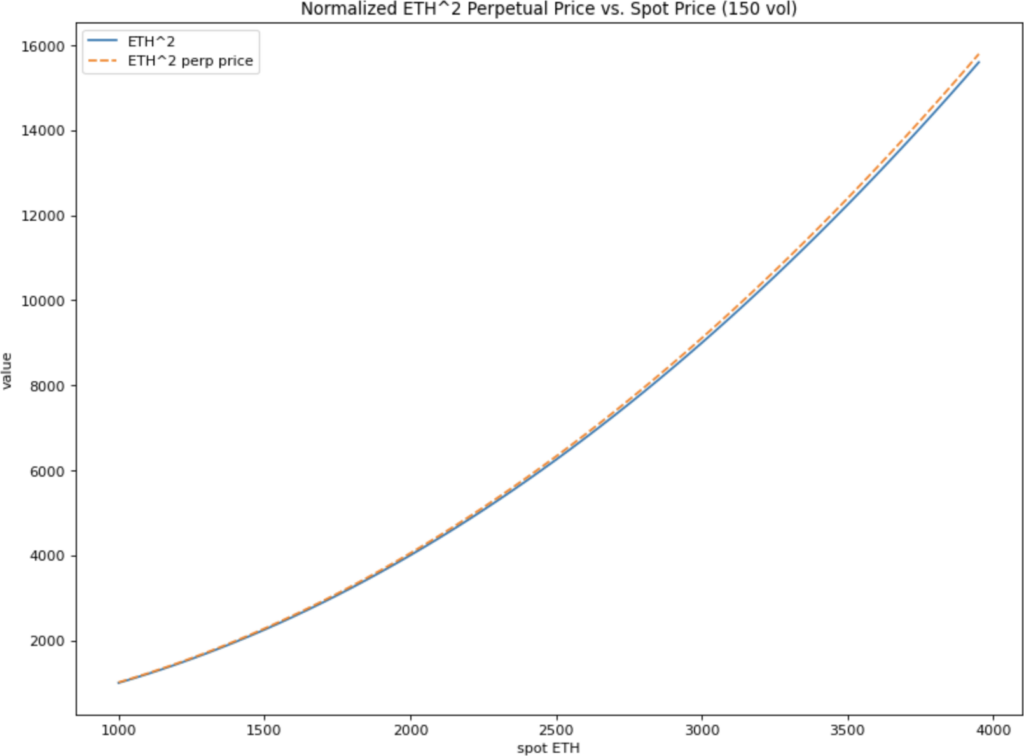
The ETH^2 power perp has a price of under Black-Scholes assumptions.

All else being equal, it will 16X as ETH 4Xs.

It has the convenient property of having a constant gamma of , meaning it offers constant optionality regardless of the price of ETH.

We affectionately call it "squeeth," short for "squared ETH."

ETH^3 Power Perp

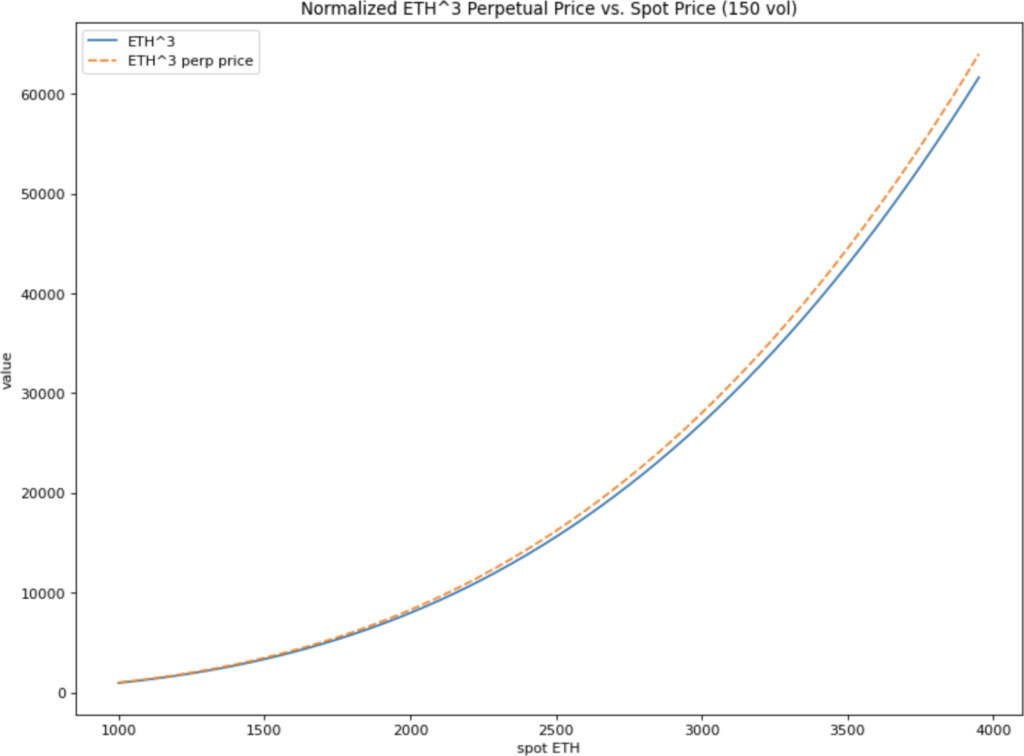
The ETH^3 power perp has a price of .

All else being equal, it will 64X as ETH 4Xs.

You can see clearly from the graph how the perp trades at a premium to its index, ETH^3, because of the optionality it provides to holders.

Python Pricing Implementation

You can see a Python implementation of power perpetuals pricing at https://github.com/para-dave/powerperps/, including a tests empirically demonstrating correctness here.

Conclusion

Power perpetuals are still in their infancy, but we have been studying them in depth since their inception and remain extremely excited about their potential.

If you are as intrigued by this new primitive as we are, we would love to hear from you. You can email dave@paradigm.xyz or DM me on Twitter, or reach out to Opyn at squeeth@opyn.co.